

The quartiles and the IQR, first off, they're easy to find and they work for any data that can put in order. Now, it may seem really counterintuitive to throw away 50% of your data and focus on the middle part, but there's some very significant advantages to that. So the middle 50% of the data has a range of four points or four units. The IQR is equal to the difference between those two values and in this dataset that's 5.5 and 1.5, we subtract the one from the other, and we get an IQR of four. We got from Q3 to Q1, and if you actually want to see it in numbers, we can do it this way. Let's go back to our data and I've grayed out the 25% on the right and the 25% on the left and now we're going to focus on just this 50% here in the middle and all we're going to do is we're going to get the range, the difference between the highest and lowest on those. In other words, it's the range of the middle 50%. The IQR is nothing more than the difference between the third quartile score, Q3, and the first quartile score, Q1.
Once you have those quartiles, you can then get something called the interquartile range, usually just called the IQR. Those five numbers define the boundaries of four regions, the four quartiles, the four quarters of the data. That's the starting point, that's the minimum score, and right here it's one. And even though it's quartiles, there actually are five of these, because you have to have Q0. Q3, the third quartile score, is at 5.5 and then when you go to the extremes, Q4, it's actually right there at 11 because that's the highest value. Q2, or the second quartile score is at 3.5.
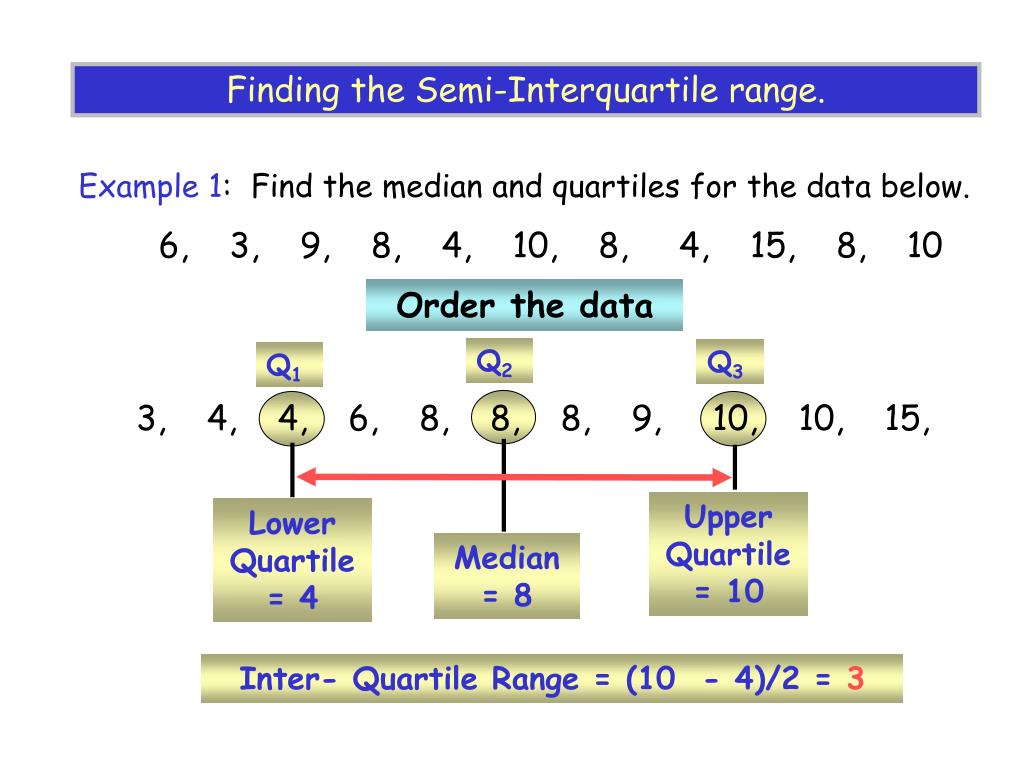
Q1 stands for quartile one, that's the first quartile score. Now we have four quarters and the quartiles are the scores that split them, it's not the actual middle of the box, it's the dividing lines, so Q1 is right here at 1.5. Split each of those boxes in half so each box contains three. Here's the data with the boxes we drew around it when we were looking for the median, where we have six on the left and six on the right. Quartiles comes from quarter because you split the data into four equal parts, four quarters. So the range, while it's very easy to do, isn't ideal, and that leads us to what may seem like a small difference, but it has a huge impact and that is the switch to something called the quartiles. And so, the range is really flexible, it works with any kind of data, as long as its quantitative or continuous, very easy to calculate, find the far right, find the far left, subtract, but the problem is it can get thrown off by outliers or any other anomalies in the data, so it also breaks down a lot. If you take our sample data here from one to 11, first you find the maximum, there it is at 11, then you find the minimum, there it is at one, and then you find the difference between the two. The range is nothing more than the difference between the maximum score and the minimum score. Let's start with the simple one, the range. The first one is the range, the second is the quartiles and something called the IQR, which stands to interquartile range, and the third one is the variance and the standard deviation, which are very, very closely related.

All of these are for a quantitative or continuous data. There are three very common measures of variability. When we talk about variability, we're talking about measures that say how spread out are the scores or how much difference is there from one observation to another. If you can only get one number for distribution, then you should use the center, but, if you can get two numbers, then the variability is the next thing to get.


 0 kommentar(er)
0 kommentar(er)
